Archive for the 'Surprises' Category

GPT-4 Fails Economics

Published
by
Steve Landsburg
on April 5, 2023
in Economics, Education, Surprises and Technology
. 106 Comments

Edit: Here is a new and improved version of the post below. I am leaving the original up for historical reasons, but I recommend following the link instead of reading this post.

*****************

Yesterday, I announced on this blog that ChatGPT had failed my economics exam. (All of the questions on this exam are taken from recent final exams in my sophomore-level course at the University of Rochester.) Multiple commenters suggested that perhaps the problem was that I was using an old version of ChatGPT.

I therefore attempted to upgrade to the state-of-the-art GPT-4, but upgrades are temporarily unavailable. Fortunately, our commenter John Faben, who has an existing subscription, offered to submit the exam for me.

The result: Whereas the older ChatGPT scored a flat zero (out of a possible 90), GPT-4 scored four points (out of the same possible 90). [I scored the 9 questions at 10 points each.] I think my students can stop worrying that their hard-won skills and knowledge will be outstripped by an AI program anytime soon.

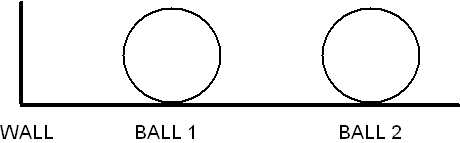
(One minor note: On the actual exams, I tend to specify demand and supply curves by drawing pictures of them. I wasn’t sure how good the AI would be at reading those pictures, so I translated them into equations for the AI’s benefit. This seems to have had no deleterious effect. The AI had no problem reading the equations; all of its errors are due to fundamental misunderstandings of basic concepts.)

Herewith the exam questions, GPT-4’s answers (in typewriter font) and the scoring (in red):

Continue reading ‘GPT-4 Fails Economics’

Print Friendly, PDF & Email
Share

Where I’ve Been

Published
by
Steve Landsburg
on April 24, 2022
in Economics, Personal, Puzzles and Surprises
. 69 Comments

A couple of weeks ago, I was in Las Vegas for the annual meeting of the Association for Private Enterprise Education, where I was honored to give an invited plenary address.

From there, I went directly to Atlanta, where I gave a short talk at the Gathering for Gardner, honoring the legacy of Martin Gardner. There were a lot of other really cool talks too.

I am sorry that the Las Vegas talk was not recorded and that the recording from the Atlanta talk won’t be available for a few months. Therefore I sat down in front of my webcam and repeated both talks, sticking as close to the original words as my memory would allow. Unfortunately there is no way to recreate the audience reaction or the question and answer periods.

Click below to view either or both of those re-creations.

The second talk is essentially a six-minute excerpt from the first. It surely benefited from the discussions here, here and here, and most especially from the comments of Bennett Haselton.

A few more words about escalators for those who care about this kind of thing:
Continue reading ‘Where I’ve Been’

Print Friendly, PDF & Email
Share

Fun Fact

Published
by
Steve Landsburg
on January 9, 2022
in Oddities and Surprises
. 5 Comments

John Tyler was born during George Washington’s administration. From 1841 to 1845 he served as the tenth president of the United States.

His grandson is alive!

Print Friendly, PDF & Email
Share

Boys, Girls and Hot Hands

Published
by
Steve Landsburg
on October 7, 2015
in Bad Reasoning, Blogging, Puzzles, Statistics and Surprises
. 64 Comments

This is a post about hot hands in basketball. But first, some relevant history:

The single most controversial topic ever broached here on The Big Questions was not Obamacare, or tax policy, or the advantages of genocide, or the policy treatment of psychic harms. It was this:

The answer, of course, is that you can’t know for sure, because (for example) by some extraordinary coincidence, the last 100,000 families in a row might have gotten boys on the first try. But in expectation, what fraction of the population is female? In other words, if there were many such countries, what fraction would you expect to observe on average?

The “official” answer — the answer, for example, that Google was apparently looking for when they posed this as an interview question — is that no stopping rule can change the fact that each birth has a 50% chance of being either male or female. Therefore the expected fraction of girls in the population is 50%.

That turns out to be wrong. It’s true that no stopping rule can change the fact that each birth has a 50% chance of being either male or female. From this it does follow that the expected number of girls is equal to the expected number of boys. But it does not follow that the expected fraction of girls in the population is 50%. Instead, that expected fraction depends on the country size, but is always less than 50%.

If you don’t see why, I encourage you to browse the archive of relevant blog posts. If you still don’t get it, I encourage you to keep browsing. Whatever your objections might be, you’ll find them addressed somewhere in the archive. I’m not interested in relitigating this. I will, however, happily renew my offer to take $5000 bets on the matter, on the terms described here. Last time around, all takers changed their minds before putting any money on the table.

Now let’s get to the hot hands.

Continue reading ‘Boys, Girls and Hot Hands’

Print Friendly, PDF & Email
Share

Follow the Bouncing Ball

Published
by
Steve Landsburg
on June 18, 2014
in Cool Stuff, Math and Surprises
. 15 Comments

If you want to compute the circumference of the observable universe to within, say, the width of a human hair, you’ll need to know about 35 digits of π, though this never seems to deter a certain sort of person from memorizing the first 100, 200 or 500 digits. But it turns out there’s no need to memorize anything at all! You can recover any number of digits you like from a simple little physics experiment that I just learned about, though it was invented over ten years ago by Professor Gregory Galperin of Eastern Illinois University. His lovely little paper is here.

To see how it works, start with two identical billiards lined up in front of a wall like so:

Now push Ball 2 toward Ball 1 and count the collisions: First Ball 2 collides with Ball 1 and pushes it toward the wall. (At this point Ball 2 has transferred all its momentum to Ball 1 and stops moving). Then Ball 1 collides with the wall and bounces back toward Ball 2. Then Ball 1 collides with Ball 2 and pushes it off to a far-away place. Three collisions. That tells you that π starts with a 3.

If you want more accuracy, make Ball 2 exactly 100 times as heavy as Ball 1. This time the sequence of events is a little more complicated, but it turns out there are exactly 31 collisons. That tells you that π starts with 3.1.

Or if you prefer, make Ball 2 exactly 10,000 times as heavy as Ball 1. You’ll get exactly 314 collisions. π starts with 3.14.

Continue reading ‘Follow the Bouncing Ball’

Print Friendly, PDF & Email
Share

IQ Test

Published
by
Steve Landsburg
on March 15, 2012
in Math and Surprises
. 39 Comments

What comes next in the following series?

(Hint: This is a calculus problem.)

Click here for the answer. Explanation forthcoming in a few days.

Click here to comment or read others’ comments.

Print Friendly, PDF & Email
Share

Uh Oh

Published
by
Steve Landsburg
on March 13, 2012
in Math, Surprises and Technology
. 45 Comments

How secure is Internet security? A team of researchers recently set out to crack the security of about 6.6 million sites around the Internet that use a supposedly “unbreakable” cryptosystem. The good news is that they succeeded only .2% of the time. The bad — and rather shocking — news — is that .2% of 6.6 million is almost 13,000. That’s 13,000 sites with effectively no security. The interesting part is what went wrong. It’s a gaping security hole that I’d never stopped to consider, but is obvious once it’s pointed out.

Continue reading ‘Uh Oh’

Print Friendly, PDF & Email
Share