Archive for the 'Logic' Category

Mamas, Don’t Let Your Babies Grow Up to Be Dollar Cost Averagers

Published
by
Steve Landsburg
on June 29, 2021
in Finance and Logic
. 22 Comments

If you’re a novice investor, looking to get into anything from the stocks to Bitcoin, someone is going to tell you that you can reduce your risk by Dollar Cost Averaging — that is, investing the same dollar amount every month. That way, this person will tell you, you are buying more of the asset when its price is low and less when it’s high.

Please do not take investment advice from this person.

I explained in The Armchair Economist why the above argument is just plain wrong, and why Dollar Cost Averaging is actually riskier than a Readjustment strategy where you invest (say) $1000 and then periodically adjust your total investment up or down as necessary to maintain its value. The logic is compelling. But the sort of people who Dollar Cost Average tend not to be the sort of people with much of an appetite for logic. So today let’s go down a different road. I’ve actually done some calculations to show you how the two strategies are likely to pan out.

Imagine a (very volatile) asset, currently selling for $1, which either doubles or halves in value each month, with equal probability. [I know, I know, the asset you’re looking to buy behaves very differently than this one. So feel free to make some other set of assumptions and re-do my calculations. You won’t get exactly the same outcomes, but you’ll probably get outcomes that are similar in spirit.]

Now let’s imagine one hundred Dollar-Cost Averagers, each investing one dollar per month for 10 months. At the end of that ten months, the average investor will be ahead by about $30. That’s not bad.

Let’s also imagine one hundred Readjusters who each invest $11.83 up front and then, at the end of each month, either withdraw or deposit funds to bring their investment right back to $11.83. Why $11.83? Because that way, these hundred Readjusters will earn, on average, exactly the same $30 that the Dollar-Cost-Averagers earn over the course of ten months.

Okay, both strategies do equally well on average. But of course, if you’re one of these investors, you probably won’t be average. So I set my computer to calculating the distribution of outcomes for each group. Here is the result, with the DCA investors on top and the Readjustment investors on the bottom:

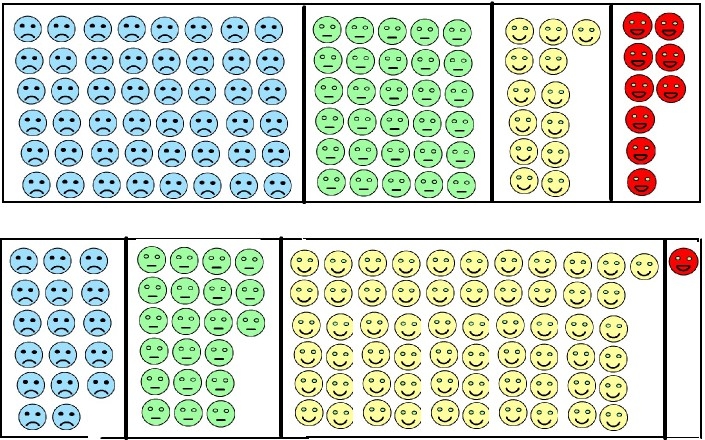
The blue guys have actually lost money. The green guys haven’t lost anything, but they’ve gained less than the average $30. The yellow guys have done better than average, and the red guys have done better still, earning over $90, which is three times the average.

Here’s the good news for the Dollar Cost Averagers: Nine of them have earned over $90, whereas only one Readjuster has earned over $90. That’s pretty much the end of the good news. In the money-losing blue category, there are far fewer Readjusters than Dollar-Cost-Averagers — about 17 versus 48. That’s right; almost half the Dollar-Cost-Averagers lose money, while only about 1/6 of the Readjusters do. 61% of the Readjusters are in the very happy yellow category, earning between $30 and $90. Only 13% of the Dollar-Cost-Averagers make it into that bracket.

Does that prove that Dollar-Cost-Averaging is inferior to Readjustment? Of course not. It all depends on what you’re looking for. Dollar-Cost-Averagers are more likely to lose money, and more likely to earn below-average returns, but in exchange, they get a 9% chance of extraordinary success while their Readjusting neighbors have only a 1% chance. Maybe that’s a gamble you want to take. That’s fine. In other words, it makes perfectly good sense to choose Dollar Cost Averaging in order to increase your risk. The guy who told you to use it to decrease your risk is still 100% wrong.

There are, of course, various real world considerations that got left out here. Different investment strategies, for example, have different tax consequences, and you might want to modify your strategy for that reason, but there’s no reason to think that after those modifications, you should be doing anything like Dollar Cost Averaging.

I want to reiterate that even before I did these calculations, I knew (at least roughly) how they were going to come out, because I trust the underlying logic (which, again, can be found in The Armchair Economist). The larger moral is that logic is trustworthy.

Click here to comment or read others’ comments.

Print Friendly, PDF & Email
Share

On Backward Induction

Published
by
Steve Landsburg
on November 12, 2018
in Economics, Humor and Logic
. 29 Comments

A Guest Post

by

William Carrington

(Note from the proprietor—I am delighted to present this guest post from my correspondent William Carrington, who might or might not have been inspired by the puzzles in Chapter Nine of Can You Outsmart an Economist?. — SL)

Like zombies and Russian spies, there are more economists among us than you might think. This can be dangerous because studies show that economists are more likely than normal people to graze their goats too long on the town commons, to rat out their co-conspirators in jailhouse interrogations, and to show up drunk on their last day at a job. This appears to be both because unethical people are drawn to economics and because economics itself teaches people to be both untrusting and untrustworthy. This feedback loop has led to the creation of famously difficult economists like John Stuart Mill and….well, it’s a long list. Like halitosis and comb-overs, the problem is worse in Washington.

Can you protect yourself against this unseen risk? Sadly, no, as economists often look all too normal and are hard to pick out from the maladjusted crowds that attend us. This is known as the identification problem in economics, and Norway’s Trygve Haavelmo was awarded a Nobel Prize for his work on this issue. Related work by Ken Arrow, also a Nobelist, proved that an infinitesimal group of economists will bollix up the welfare of an arbitrarily large population of otherwise normal people. It’s most disheartening, but I’m here to offer you a failsafe method for identifying economists. You’ll need an old refrigerator.

Continue reading ‘On Backward Induction’

Print Friendly, PDF & Email
Share

Monday Solution

Published
by
Steve Landsburg
on July 30, 2018
in Logic, Math and Puzzles
. 14 Comments

The answer to Friday’s puzzle is YES. If I am a logic machine who only states what I can prove, and if I say “If I can prove there is no God, then there is no God”, it does follow that I can prove there is no God.

Once again, it was our commenter Leo who got this first (in Friday’s comment thread, graciously rot-13’d).

As with Thursday’s solution to Wednesday’s puzzle, there are two key relevant background facts:

A) An inconsistent system can prove anything.

B) A sufficiently complex consistent system cannot prove its own consistency. (This is Godel’s second incompleteness theorem.)

Here’s the logic:

1) I’ve asserted that “if I can prove there is no God, then there is no God”. We know that I assert only things that I can prove. Therefore I can prove this assertion.

2) That means I can also prove the equivalent assertion that “if there is a God, I cannot prove otherwise”.

3) Therefore, if I take my axioms and add the axiom “There is a God”, then I can prove that there is something I cannot prove.

4) Therefore, if I take my axioms and add the axiom “There is a God”, then I can prove that my axiom system is consistent (by Background Fact A.)

5) Therefore, if I take my axioms and add the axiom “There is a God”, my axiom system is inconsistent. (Because only an inconsistent system can prove its own consistency — that is, Background Fact B.)

6) Therefore the statement “There is a God” must contradict my axiom system.

7) This can happen only if my axiom system is able to prove that “There is no God”.

So yes, I can prove there is no God.

Continue reading ‘Monday Solution’

Print Friendly, PDF & Email
Share

Flashback

Published
by
Steve Landsburg
on April 23, 2014
in Logic, Math, Ontology and Philosophy
. 0 Comments

Last week, I posted video of my talk to the undergraduate math students on truth, provability and the fabric of the universe — and heard from several readers who requested that I post it in a non-flash format.

My readers’ wish is my command. Here is the file for download in an m4v format.

This is relatively low resolution. The best viewing is still the flash version here.

Click here to comment or read others’ comments.

Print Friendly, PDF & Email
Share

Truth, Provability and the Fabric of the Universe

Published
by
Steve Landsburg
on April 16, 2014
in Logic, Math, Ontology and Physics
. 18 Comments

Here is my talk to the University of Rochester’s Society of Undergraduate Math Students on “Truth, Provability and the Fabric of the Universe”. The audience was great, and except for a couple of slips of the tongue (like “Sir William of Ockham” for “William of Ockham”), I thought it went very well.

Faithful readers will recognize multiple themes from the book The Big Questions, and from numerous past blog posts, including:

Continue reading ‘Truth, Provability and the Fabric of the Universe’

Print Friendly, PDF & Email
Share

Hard and Harder

Published
by
Steve Landsburg
on July 5, 2013
in Logic and Puzzles
. 14 Comments

If you failed to solve Wednesday’s problem on Knights, Knaves and Crazies, take comfort from the fact that this has circulated among philosophers under the title “The Hardest Logic Problem Ever”. MIT philosopher George Boolos discussed it in the Harvard Review of Philosophy back in 1996. In that version, Crazies are never silent. But Oxford philosopher Gabriel Uzquiano soon observed that this can’t be the hardest logic problem ever, because it gets harder if the Crazies can be silent. Uzquiano’s new “hardest logic problem ever” was solved by the philosophers Gregory Wheeler and Pedro Barahona — and then solved again, substantially more elegantly, I think, in Wednesday’s comments section right here.

A few more thoughts, on the problem, its solution, and how to make it harder:

Continue reading ‘Hard and Harder’

Print Friendly, PDF & Email
Share

Knights, Knaves and Crazies

Published
by
Steve Landsburg
on July 3, 2013
in Logic and Puzzles
. 97 Comments

SmullyanThe best dozen or so puzzle books ever written are, without a doubt, the works of Raymond Smullyan. If you’ve never encountered these, stop right now and order yourself a copy of What is the Name of This Book?, which is brilliant on multiple levels. On the surface, it’s a book of particularly amusing little brain teasers. One level down, those brain teasers contain a proof of Godel’s Incompleteness Theorem — solve all the riddles and you’ll have painlessly understood the proof!

Smullyan’s books are heavily populated by Knights who always tell the truth, Knaves who always lie, and bewildered travelers trying to distinguish one from the other via their cryptic utterances. Today’s puzzle is Smullyan-like in its set-up but considerably more difficult than most. It’s been proposed and discussed in philosophy journals, but I’m suppressing the sources (and rewording the problem) to make it a little harder to Google. I’ll of course pay appropriate homage to the authors when I post solutions in the near future. Meanwhile, if you’ve seen this before, or if you’ve found the answer on line, please restrain yourself from posting spoilers. But do post whatever you manage to come up with on your own.

And now to the puzzle:

Continue reading ‘Knights, Knaves and Crazies’

Print Friendly, PDF & Email
Share

Accounting for Numbers

Published
by
Steve Landsburg
on November 14, 2012
in Logic, Math, Ontology and Philosophy
. 81 Comments

Over at Less Wrong, the estimable Eliezer Yudkowsky attempts to account for the meaning of statements in arithmetic and the ontological status of numbers. I started to post a comment, but it got long enough that I’ve turned my comment into a blog post. I’ve tried to summarize my understanding of Yudkowsky’s position along the way, but of course it’s possible I’ve gotten something wrong.

It’s worth noting that every single point below is something I’ve blogged about before. At the moment I’m too lazy to insert links to all those earlier blog posts, but I might come back and put the links in later. In any event, I think this post stands alone. Because it got long, I’ve inserted section numbers for the convenience of commenters who might want to refer to particular passages.

1. Yudkowsky poses, in essence, the following question:

Main Question, My Version: In what sense is the sentence “two plus two equals four” meaningful and/or true?

Yudkowsky phrases the question a little differently. What he actually asks is:

Main Question, Original Version: In what sense is the sentence “2 + 2 = 4″ meaningful and/or true?”

This, I think, threatens to confuse the issue. It’s important to distinguish between the numeral “2”, which is a formal symbol designed to be manipulated according to formal rules, and the noun “two”, which appears to name something, namely a particular number. Because Yudkowsky is asking about meaning and truth, I presume it is the noun, and not the symbol, that he intends to mention. So I’ll stick with my version, and translate his remarks accordingly.

2. Yudkowsky provisionally offers the following answer:

Continue reading ‘Accounting for Numbers’

Print Friendly, PDF & Email
Share

Simple as ABC

Published
by
Steve Landsburg
on September 13, 2012
in Logic, Math, Ontology and Philosophy
. 46 Comments

The (really really) big news in the math world today is that Shin Mochizuki has (plausibly) claimed to have solved the ABC problem, which in turn suffices to settle many of the most vexing outstanding problems in arithmetic. Mochizuki’s work rests on so many radically new ideas that it will take the experts a long time to digest. I, who am not an expert, will surely die with only a vague sense of the argument. But based on my extremely limited (and possibly mistaken) understanding, it appears that Mochizuki’s breakthrough depends at least partly on his willingness to abandon the usual axioms for the foundations of mathematics and replace them with new axioms. (See, for example, the first page of these notes from one of Mochizuki’s lectures. You can find other related notes here.)

That’s interesting for a lot of reasons, but the one that’s most topical for The Big Questions is this: No mathematician would consider rejecting Mochizuki’s proof just because it relies on new axiomatic foundations. That’s because mathematicians (or at least the sort of mathematicians who study arithmetic) don’t particularly care about axioms; they care about truth.

There’s a widespread misconception that arithmetic is about “what can be derived from the axioms”, which is a lot like saying that astronomy is about “what can be discovered through telescopes”. Axiomatic systems, like telescopes, are investigative tools, which we are free to jettison when better tools come along. The blather of thoughtless imbeciles notwithstanding, what really matters is the fundamental object of study, whether it’s the system of natural numbers or the planet Jupiter.

Mathematicians care about what’s true, not about what’s provable; if a truth isn’t provable, we’re fine with changing the rules of the game to make it provable.

Continue reading ‘Simple as ABC’

Print Friendly, PDF & Email
Share

The Number Devil

Published
by
Steve Landsburg
on May 2, 2012
in Logic, Math, Ontology and Philosophy
. 42 Comments

devilIn the comments section of Bob Murphy’s blog, I was asked (in effect) why I insist on the objective reality of the natural numbers (that is, the counting numbers 0,1,2,3…) but not of, say, the real numbers (that is, the numbers we use to represent lengths — and that are themselves represented by possibly infinite decimal expansions).

There seem to be two kinds of people in the world: Those with enough techncal backgroud that they already know the answer, and those with less technical background, who have no hope — at least without a lot of work — of grasping the answer. I’m going to attempt to bridge that gap here. That means I’m going to throw a certain amount of precision to the winds, in hopes of being comprehensible to a wider audience.

Continue reading ‘The Number Devil’

Print Friendly, PDF & Email
Share

That Does Not Compute

Published
by
Steve Landsburg
on April 26, 2012
in Logic, Math, Ontology and Philosophy
. 128 Comments

stanleyStanley Tennenbaum was an itinerant mathematician with, for much of his adult life, no fixed address and no permanent source of income. Sometimes he slept on park benches. He didn’t have a lot of teeth.

But if you were involved with mathematics in the second half of the twentieth century, sooner or later you were going to cross paths with Stanley, probably near the coffee machine in a math department. He’d proudly show you the little book he carried in his breast pocket, with the list of people to whom he owed money. Then he’d teach you something, or he’d tell you a good story.

Stanley had little tolerance for convention. His one permanent job, at the University of Rochester, came to an abrupt end during a faculty meeting where he spit on the shoes of the University president and walked out. Surely the same personality trait had something to do with his departure from the University of Chicago without a Ph.D., though the paper he wrote there (at age 22) has acquired fame and influence far beyond many of the doctoral theses of his more conventionally successful classmates. I’d like to tell you a little about that paper and what I think it means for the foundations of mathematics.

Continue reading ‘That Does Not Compute’

Print Friendly, PDF & Email
Share

The Big Surprise

Published
by
Steve Landsburg
on December 15, 2011
in Logic, Math and Puzzles
. 26 Comments

Back in the 1930’s, Kurt Godel proved two amazing facts about arithmetic: First, there are true statements in arithmetic that can’t be proven. Second, the consistency of arithmetic can’t be proven (at least not without recourse to logical methods that are on shakier ground than arithmetic itself).

Yesterday, I showed you Gregory Chaitin’s remarkably simple proof, of Godel’s first theorem. Today, I’ll show you Shira Kritchman and Ron Raz’s remarkably simple (and very recent) proof of Godel’s second theorem. If you work through this argument, you will, I think, have no trouble seeing how it was inspired by the paradox of the surprise examination.

Continue reading ‘The Big Surprise’

Print Friendly, PDF & Email
Share

Berry Interesting

Published
by
Steve Landsburg
on December 14, 2011
in Logic, Math and Puzzles
. 37 Comments

confiture and ingredientsToday, I’m going to give you a short, simple proof of Godel’s First Incompleteness Theorem — the one that says there are true statements in arithmetic that can’t be proven. The proof is due to Gregory Chaitin, and it is far far simpler than Godel’s original proof. A bright high-schooler can grasp it instantly. And it’s wonderfully concrete. At the end, we’ll have an infinite list of statements, all easy to understand, and none of them provable — but almost all of them true (though we won’t know which ones).

Continue reading ‘Berry Interesting’

Print Friendly, PDF & Email
Share

A Tale of Three Paradoxes

Published
by
Steve Landsburg
on December 13, 2011
in Logic, Math and Puzzles
. 20 Comments

This is a tale of three paradoxes and why they matter.

  • First, the ancient Liar Paradox: “This sentence is false”. If this sentence is true, it must be false. If it’s false, it must be true.
  • Next, the century-old Berry Paradox: Call a phrase “short” if it contains fewer than 13 words. The English language contains a finite number of words, and hence a finite number of short phrases. Hence there must be some natural numbers that can’t be described by any short phrase. Among these natural numbers, there must be a smallest. What is that natural number? Why, it’s the smallest natural number that can’t be described by any short phrase, of course. Except that this number is in fact described by the short phrase in boldface.
  • Finally, the more modern Paradox of the Surprise Examination (or the Unexpected Hanging), which we discussed yesterday.

The paradoxes are slippery, because they are stated in the imprecise language of English. But each of them has inspired a precise mathematical counterpart that is central to a brilliant argument in mathematical logic.

Continue reading ‘A Tale of Three Paradoxes’

Print Friendly, PDF & Email
Share

The Surprise Exam, and More Surprises

Published
by
Steve Landsburg
on December 12, 2011
in Logic, Math and Puzzles
. 60 Comments

surpriseexamIf you’re the sort of person who reads this blog, you’re likely to be familiar with the paradox of the unexpected hanging, which has been floating around since 1943 but achieved popular notoriety around 1969 through the writing of Martin Gardner. But you’re less likely to be aware that the unexpected hanging plays a central role in a wonderful new piece of serious mathematics related to algorithmic complexity, Godel’s theorems, and the gap between truth and provability.

The unexpected hanging might as well be a surprise examination, and that’s the form in which I present this paradox to my students every year: In a class that meets every weekday morning, the professor announces that there will be an exam one day next week, but that students won’t know exactly which day until the exams are handed out.

The students, of course, immediately start trying to guess the day of the exam. One student (call him Bob) observes that the quiz can’t be on Friday — because if it is, the students will know that by Thursday afternoon. After all, if Monday, Tuesday, Wednesday and Thursday mornings have all passed by, only Friday remains. A Friday exam can’t be a surprise exam.

A more thoughtful student (call her Carol) observes that this means the quiz must be on one of Monday, Tuesday, Wednesday or Thursday — and that if it’s on Thursday, they’ll know that by Wednesday night. After all, Friday’s ruled out, so if Monday, Tuesday and Wednesday have passed by, then only Thursday remains. That rules out a surprise exam on Thursday.

Another student (call him Ted) observes that thanks to Bob and Carol, we know the exam must be on one of the first three days of the week — which means that if it’s not on Monday or Tuesday, it must be on Wednesday. Therefore if it’s on Wednesday, they’ll know this by Tuesday night. Scratch Wednesday from the list of possibilities.

Now Ted’s girlfriend Alice points out that the exam can’t be on Tuesday either. Whereupon Bob concludes that the exam must be on Monday. But wait a minute! Carol points out that if they know the exam will be on Monday, it can’t be a surprise. Therefore no surprise exam is possible.

The students, relieved, decide not to study. But they’re awfully surprised when they show up in class the following Tuesday and the professor hands out an exam.

Continue reading ‘The Surprise Exam, and More Surprises’

Print Friendly, PDF & Email
Share

Big News

Published
by
Steve Landsburg
on October 4, 2011
in Logic, Math, Progress and Truthseeking
. 53 Comments

Last week, the highly distinguished Princeton Professor Ed Nelson announced a proof that the Peano axioms for arithmetic are inconsistent — and hence so is arithmetic itself. If true, this would be much bigger news than faster-than-light neutrinos. It would be bigger news than a discovery that the South had won the American Civil War. It would be far, far bigger news than a discovery that all life on Earth was intelligently designed.

There are, after all, multiple proofs that Peano Arithmetic (that is, the fragment of arithmetic described by the Peano axioms) is consistent. Among those, the simplest and most convincing (to the overwhelming majority of mathematicians) is this: The axioms of Peano Arithmetic, and therefore the theorems of Peano Arithmetic, are all true statements about the natural numbers — and a set of true statements cannot contradict itself.

Ed Nelson rejects that argument because (exempting himself from that overwhelming majority) he doesn’t believe in the set of natural numbers — or perhaps even in individual numbers when those numbers are very large. (How do you know that 810000 exists? Have you ever counted to it?)

Continue reading ‘Big News’

Print Friendly, PDF & Email
Share

Friday Humor

Published
by
Steve Landsburg
on August 5, 2011
in Humor and Logic
. 15 Comments

Three logicians walk into a bar.

The bartender says: “Would any of you guys like a drink?”

The first logician says: “I don’t know.”

The second logician says: “I don’t know.”

The third logician says: “No.”

Hat tip to Adam Merberg, who isn’t sure of the source.

Click here to comment or read others’ comments.

Print Friendly, PDF & Email
Share

Inconsistency

Published
by
Steve Landsburg
on June 8, 2011
in Logic and Math
. 75 Comments

voevodsky-80thVladimir Voevodsky, one of the world’s best and most influential mathematicians, has stirred up a bit of a hornet’s nest with a video lecture suggesting the possibility that the Peano Axioms — the standard axioms for arithmetic — might be inconsistent.

Since the Peano Axioms are known to be consistent, it’s tempting to dismiss the whole lecture as either a prank or a shocking display of ignorance. The latter temptation is buttressed somewhat by Voevodsky’s bold misstatement of Godel’s Incompleteness Theorem, which plays a central role in the lecture. On the other hand, Voevodsky is smarter than almost anyone else on earth, which earns him the benefit of the doubt — maybe what he’s saying is subtler than it seems. On the other hand, some of those in the “shocking display of ignorance” camp are among the few people in the world who might be as smart as Voevodsky.

To believe that the Peano Axioms are inconsistent, Voevodsky must reject all of the known proofs that they are consistent. In particular, he must reject the simplest and most convincing of all those proofs, which goes like this:

  1. The Peano Axioms, and therefore all of their logical consequences, are true statements about the natural numbers,
  2. A collection of true statements cannot contradict itself.
  3. QED.

Continue reading ‘Inconsistency’

Print Friendly, PDF & Email
Share

Lord Russell’s Nightmare

Published
by
Steve Landsburg
on December 20, 2010
in Anniversaries, Heroes, Logic, Math and Philosophy
. 32 Comments

bertieBertrand Russell, that most rational of men, was nonetheless plagued by intermittent depression and the occasional nightmare. Including this one, as reported by Russell’s confidante, the mathematician G.H. Hardy:

[Russell] was in the top floor of the University Library, about A.D. 2100. A library assistant was going round the shelves carrying an enormous bucket, taking down books, glancing at them, restoring them to the shelves or dumping them into the bucket. At last he came to three large volumes which Russell could recognize as the last surviving copy of Principia Mathematica. He took down one of the volumes, turned over a few pages, seemed puzzled for a moment by the curious symbolism, closed the volume, balanced it in his hand and hesitated….

Principia Mathematica, to which Russell had devoted ten years of his life, was his (and co-author Alfred North Whitehead‘s) audacious and ultimately futile attempt to reduce all of mathematics to pure logic. It is a failure that enabled some of the great successes of 20th century mathematics. And — the first volume having been published in December, 1910 — this is its 100th birthday.

Continue reading ‘Lord Russell’s Nightmare’

Print Friendly, PDF & Email
Share

Eighty Years of Incompleteness

Published
by
Steve Landsburg
on October 25, 2010
in Anniversaries, Logic and Math
. 9 Comments

godelThis weekend marked the 80th anniversary of the most significant event in the history of logic since the days of Aristotle. On October 23, 1930, the 24-year-old Kurt Godel presented his incompleteness theorems to the Vienna Academy of Sciences.

The first incompleteness theorem says this: No matter what axioms you start with, there will always be statements in arithmetic that you can neither prove nor disprove. (I am glossing over some technicalities here, but in this context they are not important.) Some of those statements take the form “Such-and-such an equation has (or does not have) any solutions”. You tell me your axioms, and I’ll produce an equation that you can neither prove solvable nor prove insolvable.

Continue reading ‘Eighty Years of Incompleteness’

Print Friendly, PDF & Email
Share

Basic Arithmetic, Part III: The Map is Not the Territory

Published
by
Steve Landsburg
on August 19, 2010
in Logic and Math
. 35 Comments

Today let’s talk about consistency.

Suppose I show you a map of Nebraska, with as-the-crow-flies distances marked between the major cities. Omaha to Lincoln, 100 miles. Lincoln to Grand Island, 100 miles. Omaha to Grand Island, 400 miles.

You are entitled to say “Hey, wait a minute! This map is inconsistent. The numbers don’t add up. If it’s 400 miles straight from Omaha to Grand Island, then there can’t be a 200 mile route that goes through Lincoln!”

So a map can be inconsistent. (It can also be consistent but wrong.) Nebraska itself, however, can no more be inconsistent than the color red can be made of terrycloth. (Red things can be made of terrycloth, but the color red certainly can’t.)

With that in mind, suppose I give you a theory of the natural numbers — that is, a list of axioms about them. You might examine my axioms and say “Hey! These axioms are inconsistent. I can use them to prove that 0 equals 1 and I can also use them to prove that 0 does not equal 1!” And, depending on the theory I gave you, you might be right. So a theory can be inconsistent. But the intended model of that theory — the natural numbers themselves — can no more be inconsistent than Nebraska can. Inconsistency in this context applies to theories, like the Peano axioms for arithmetic, not to structures, like the natural numbers themselves.

Continue reading ‘Basic Arithmetic, Part III: The Map is Not the Territory’

Print Friendly, PDF & Email
Share

Basic Arithmetic, Part II

Published
by
Steve Landsburg
on August 18, 2010
in Logic and Math
. 40 Comments

Today’s mini-lesson in the foundations of mathematics is about the key distinction between theories and models.

The first thing to keep in mind is that mathematics is not economics, and therefore the vocabulary is not the same. In economics, a “model” is some sort of an approximation to reality. In mathematics, the word model refers to the reality itself, whereas a theory is a sort of approximation to that reality.

A theory is a list of axioms. (I am slightly oversimplifying, but not in any way that will be important here.) Let’s take an example. I have a theory with two axioms. The first axiom is “Socrates is a man” and the second is “All men are mortal”. From these axioms I can deduce some theorems, like “Socrates is mortal”.

That’s the theory. My intended model for this theory is the real world, where “man” means man, “Socrates” means that ancient Greek guy named Socrates, and “mortal” means “bound to die”.

But this theory also has models I never intended. Another model is the universe of Disney cartoons, where we interpret “man” to mean “mouse”, we interpret “Socrates” to mean “Mickey” and we interpret “mortal” to mean “large-eared”. Under that interpretation, my axioms are still true — all mice are large-eared, and Mickey is a mouse — so my theorem “Socrates is mortal” (which now means “Mickey is large-eared”) is also true.

Continue reading ‘Basic Arithmetic, Part II’

Print Friendly, PDF & Email
Share

Godel, Fermat, Hercules

Published
by
Steve Landsburg
on July 28, 2010
in Logic and Math
. 41 Comments

HerculesAndHydraYesterday I answered one of Coupon Clipper‘s questions about Godel’s Theorem. Today I’ll tackle the other: Does Godel’s Theorem matter on a day-to-day basis to practicing mathematicians?

And the answer is: Of course not. Mathematicians care about what’s true, not about what’s provable from some arbitrary set of axioms. (Of course this is an overgeneralization; some mathematicians have built distinguished careers on worrying about what’s provable from various sets of axioms. But they are a small minority.) Godel’s Theorem says that not all true things are provable. But for the most part, we’re happy just to know they’re true.

The flashiest example I can give you—and one I’ve used on this blog before—is Fermat’s Last Theorem, which says that no equation of the form xn + yn = zn has any solutions, as long as n is at least 3 and x, y and z are non-zero. Proving this was the was most famous unsolved problem in mathematics for 350 years until it was solved (to much public fanfare) by Frey, Serre, Ribet and Wiles in the 1980’s and 1990’s.

We know from that work that Fermat’s Last Theorem is true. However, we still don’t know whether Fermat’s Last Theorem follows from the standard axioms for arithmetic. And—this is the point—very few mathematicians care very much, at least by comparison to how much they care about the theorem itself. (Here is one of my favorite papers on the subject. Tellingly, the author is a philosopher.)

Continue reading ‘Godel, Fermat, Hercules’

Print Friendly, PDF & Email
Share

First Things and Second Things

Published
by
Steve Landsburg
on July 27, 2010
in Logic and Math
. 21 Comments

The occasional commenter who goes by the name Coupon Clipper has emailed me some interesting questions about Godel’s Theorem. I think I’ll answer them here.

The first question is about first-order versus second-order logic, so let me explain the distinction. When we reason formally about arithmetic, we need to clearly specify the ground rules. This means, among other things, specifying the language and grammar we’re allowed to use. A very simple language might allow us to say things like “2 + 3 = 5” or “8 is an even number”. With a language like that, you could talk about a lot of sixth grade arithmetic, but you wouldn’t be able to say anything very interesting beyond that. To talk about the questions mathematicians care about, you need a language that contains words like “every”, as in Every number can be factored into primes or Every number can be written as a sum of four squares or Every choice of positiive numbers x, y, and z will yield a non-solution to the equation x3+y3=z3 . That language is called first-order logic.

With first order logic we can specify a set of axioms, and then follow a prescribed set of rules to deduce consequences. For example, if you’ve already established that every number is a sum of four squares, then you’re allowed to conclude that 1,245,783 is a sum of four squares. (The general rule is that if you’ve proved that every number has some particular property, then you’re allowed to conclude that any particular number has that property.)

Continue reading ‘First Things and Second Things’

Print Friendly, PDF & Email
Share

Just the Facts

Published
by
Steve Landsburg
on April 28, 2010
in Logic, Math and Ontology
. 65 Comments

jackwebbDuring our brief intermission last week, commenters chose to revisit the question of whether arithmetic is invented or discovered—a topic we’d discussed here and here. This reminded me that I’ve been meaning to highlight an elementary error that comes up a lot in this kind of discussion.

It is frequently asserted that the facts of arithmetic are either “tautologous” or “true by definition” or “logical consequences of the axioms”. Those are three different assertions, and all of them are false. (This is not a controversial statement.)

The arguments made to support these assertions are not subtly flawed; they are overtly ludicrous. Almost always, they consist of “proof by example”, as in “1+1=2 is true by definition; therefore all the facts of arithmetic are true by definition”. Of course one expects to stumble across this sort of “reasoning” on the Internet, but it’s always jarring to see it coming from people who profess an interest in mathematical logic. (I will refrain from naming the worst offenders.)

So let’s consider a few facts of arithmetic:

Continue reading ‘Just the Facts’

Print Friendly, PDF & Email
Share

The Self-Referential Test

Published
by
Steve Landsburg
on December 22, 2009
in Logic and Puzzles
. 7 Comments

This quiz amused the hell out of me. I hope it does the same for you.

Edited to add: In comments, Mike H points me (and you) to this even better quiz, which seems to have been the model for the one I linked to. Enjoy your day.

Print Friendly, PDF & Email
Share

Non-Simple Arithmetic

Published
by
Steve Landsburg
on December 17, 2009
in Logic, Math, Ontology and Philosophy
. 87 Comments

complexThe Intelligent Design folk tell you that complexity requires a designer.

The Richard Dawkins crowd tell you that complexity must evolve from simplicity.

I claim they’re both wrong, because the natural numbers, together with the operations of arithmetic, are fantastically complex, but were neither created nor evolved.

I’ve made this argument multiple times, in The Big Questions, on this blog, and elsewhere. Today, I aim to explain a little more deeply why I say that the natural numbers are fantastically complex.

Continue reading ‘Non-Simple Arithmetic’

Print Friendly, PDF & Email
Share

Trading Up

Published
by
Steve Landsburg
on November 11, 2009
in Economics, Logic and Policy
. 46 Comments

A reader has just emailed me a link to a Washington Post story about North Carolina workers losing their jobs to foreign competition. Presumably he believes there’s a larger moral here, because his subject line is “Wrong again, Steve”. Here is a slightly edited version of my emailed response:

It would be dishonest for me or anyone else to defend free trade by pointing to its advantages while ignoring its disadvantages.

It is equally dishonest to oppose free trade by pointing to its disadvantages while ignoring its advantages.

What you need is a framework that accounts for all the advantages and disadvantages, together with enough of a logical structure to instill confidence that nothing imporant has been overlooked. Thats what economic theory supplies. You can find that theory in the economics textbooks. You can also find (I think) a pretty good summary of it in The Big Questions.

My correspondent wrote back with a pointer to a website with fifty years of what he calls “extrapolatable stats” that he thinks supply the necessary framework. This misses the point entirely. There is no way a hodgepodge of numbers can settle the question of whether something’s been left out. For that you need a theory.

Continue reading ‘Trading Up’

Print Friendly, PDF & Email
Share