 |
 |
 |
 |
 |
 |
You and a stranger have been instructed to meet up sometime tomorrow, somewhere in New York City. You (and the stranger) can decide for yourselves when and where to look for each other. But there can be no advance communication. Where do you go?
Me, I’d be at the front entrance to the Empire State Building at noon, possibly missing my counterpart, who might be under the clock at Grand Central Station. But, because there are only a small number of points in New York City that stand out as “extra-special”, we’ve at least got a chance to find each other.
A Schelling point is something that stands out from the background so sharply that we can expect people to coordinate around it. Schelling points are on my mind this week, because I’ve just heard David Friedman give a fascinating talk about the evolution of property rights, and Schelling points play a big role in his story. But that story is not the topic of this post.
Instead, I’m curious about the Schelling points that say, two mathematicians, or two economists, or two philosophers, or two poets, or two street hustlers might converge on. Suppose, for example, that you asked two mathematicians each to separately pick a number between 200 and 300, with a prize if their answers coincide. I’m guessing they both go for 256, the only power of two within range.
What about, say, numbers between 1000 and 2000? Historians might converge on 1066; (American) accountants on 1040. But for mathematicians, the obvious Schelling point would be 1729, the smallest number that can be written as the sum of two cubes in two different ways. (It’s 93+103, but it’s also 123+13). The reason we’re likely to jump at this number is that we were all brought up on the famous story of G.H. Hardy‘s visit to Ramanujan‘s deathbed:
Hardy used to visit him, as he lay dying in hospital at Putney. It was on one of those visits that there happened the incident of the taxicab number. Hardy had gone out to Putney by taxi, as usual his chosen method of conveyance. He went into the room where Ramanujan was lying. Hardy, always inept about introducing a conversation, said, probably without a greeting, and certainly as his first remark: ‘I thought the number of my taxicab was 1729. It seemed to me rather a dull number.’ To which Ramanujan replied: ‘No, Hardy! No, Hardy! It is a very interesting number. It is the smallest number expressible as the sum of two cubes in two different ways.
(This version is from C.P. Snow‘s introduction to Hardy’s brief autobiography A Mathematician’s Apology. If you’ve never read this book, you should not read another thing until you’ve filled this gap — and I envy you the magical experience you’re about to have!)
In a somewhat higher range, the clear Schelling point is 196883. This one’s a little harder to explain, but here’s a brief (and necessarily imperfect) overview: One of the great accomplishments of the 1970s and 1980s was a complete classification of all the possible ways in which an object can be symmetric. (Actually, I’ve already oversimplified; the classification covers only the “simplest” kinds of symmetries, which in turn are known to be the building blocks of all other symmetries. There are still plenty of open questions about how many ways those building blocks can be joined together).
Most of these symmetries fit into natural families (like the family consisting of the symmetries of a triangle, the symmetries of a square, the symmetries of a pentagon and so on). But a few stand alone, and the one that stands most strikingly alone is the so-called “monster” symmetry. If you live in three or four dimensions like the rest of us, then you’ve never seen an object with this kind of symmetry, because it first manifests itself in a space with 196,883 dimensions.
Now let’s forget about symmetry for a moment and talk about something else entirely. One of the most important functions in number theory is the “elliptic modular function”, which starts out like this:
Notice the 196884? Notice how close it is to 196883? Sort of a curious coincidence, no?
Here’s another coincidence: 21493760 is 196884 + 21296876. Guess what’s the next higher dimension in which the monster symmetry occurs? It’s 21296876.
And on it goes. The next term in the elliptic modular function is 864299970 q3 And we can write 86429970 = 842609326 + 21296876 + 196883 + 196883 + 2. Except for that 2, the numbers on the right are all dimensions in which the monster symmetry occurs. There are similar suggestive patterns for all the other coefficients of the elliptic modular function.
These “coincidences” are so extraordinary that they can’t possibly be coincidences, but tracking down their sources turned out to be one of the great adventures of 20th century mathematics. The hero of that quest was Richard Borcherds who managed to figure out what was going on using, of all things, ideas that come from string theory, a branch of mathematical physics that has, on the surface, as little to do with monster symmetries and the elliptic modular function as they have to do with one another.
All of which goes to explain why I sometimes wear a t-shirt that says
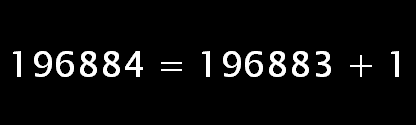
Not many people understand it, but when someone does, we know instantly that we have something in common.
What are the Schelling points for the people who share your hobby or profession? What numbers, places, or things could you count on a fellow practitioner to see as the “obvious” choices?



Well probably the most prevalent in the self styled nerd community would be 42.
@1
Also couldn’t help thinking 1337 for Steve’s second example.
“Suppose, for example, that you asked two mathematicians each to separately pick a number between 200 and 300, with a prize if their answers coincide. I’m guessing they both go for 256, the only power of two within range.”
I don’t know how common the knowledge that “256 is the only power of two within range” is among mathematicians, but if you actually asked people to do this (even if they are all mathematicians) I think 250 would be more common, are you honestly saying you would pick 256 if you were actually participating in this contest?
Whenever I have presumed that someone else would have homed in on what I considerd to be a Schelling point they have said something like “why on Earth would you have thought that?”
Your picking numbers is a Schelling point for you. Shakespeare scholars would congregate either at Stratford or the Globe Theatre. James Bond fans would all select Vodka Martini (shaken, not stirred) as a drink.
Your number between 200 and 300, I immediately thought of 250 as the only point equally between the two extremes. 256 might have been second, as I had started to think about computer memory, the multiples of which are powers of 2 and with which we are familiar. Of course I am not a mathematician.
Most numbers seem magical to me. If you have never tried looking up numbers in wikipedia, try it now. An example that caught my fancy is this one : http://en.wikipedia.org/wiki/666_(number)#In_mathematics
For physicists, 137 is an obvious choice.
I once worked on a book of baseball statistical studies. The cover was going to have a picture of a calculator (among other things). What number should be on the calculator? The best we could come up with was 714.
The cover got changed to something else, but I was still surprised how hard it was to come up with a number that represents baseball, other than “9”.
73 is another Schelling (and Sheldon) point.
This reminds me of a Poul Anderson SciFi novel, in which a small number of people are found to have the ability to travel in time. Someone wants to round them all up, so he sends a few people with this ability back to the time that he thinks will attract the most people – the Crusifixion.
This presents an interesting question, similar to Steve’s: if you could observe, in person, one event in world history, which would you choose? Probably most of the important events of history were undramatic at the time – except of course for wars.
How about Kaprekar’s constant, 6174? In general, a number that has a name would be a good choice.
The meaning of the phrase ‘to second-guess [someone]’ in the variety of English I speak seems to encapsulate at least a part of this Schelling point idea. What’s interesting about the phrase is the element of (something like) reflexivity.
But some online dictionaries give another, simpler meaning as the main one. Phrases like this often have their meanings shift to something simpler (e.g. the way ‘begs the question’ is used by journalists to mean simply ‘raises the question’). Is this phenomenon related to Schelling points, I wonder?
I see the concept is in fact used in linguistics. It’s obviously relevant to pidgins and creoles.
I honest to goodness did pick 256 and 1729, so Steve and I win something. Did not pick 196883. Would probably have picked 2^18 in that kind of range.
187
For those of us in the thug life.
Chuuuch.
(Think gin n’ juice, if this makes no sense to any of you).
If you had to try to get the same number between 1 and 100 it is difficult as there are so many claims. I had to look up the 73 reference, although I had seen that episode. I don’t think it has anywhere near the recognition as 42 even among nerds.
For someone that loves beans or ketchup 57 springs to mind. Apparently 37 is the most commonly chosen number if people are asked to choose a random number between 1 and 100. For those with a differen inclination 69 might be obvious.
@Phil #7, I would have chosen .39955, Ted Williams’s batting average prior to the final day of the 1941 season. Or .406, his average after that day.
Potheads would go for 420.
Andy at #3:
You and I both would have won the prize. What do we have in common? We are the type of people that like to win prizes instead of signaling faux intellecualism via nerdy trivia.
Two jazz musicians who could win a prize for simultaneously playing the same tune at the end of a four-count would probably choose Coltrane’s “Giant Steps.”
As a general rule, if the parameters of the request are to pick a number between x and y, inclusive, so as to have the highest probability of someone else picking the same number, I would pick x.
For example, “Choose a number between 1 and 1,000,000, inclusive.” I would choose 1.
P.S. Youtube video that explains that if you ask a person to choose a random number between 1 and 10, about 45% of the time he or she will choose 7: http://www.youtube.com/watch?v=SxP30euw3-0
I second Tristan. I have in fact played the game pick a number with people who pick 42. If you don’t get the reference take a hike :)
If you are a Toronto Maple Leaf fan, and you know I am a Toronto Maple Leaf fan, what number do you choose?
I’ve found this idea fascinating for ages. My usual way of posing the question in Steve’s introduction is “you and a complete stranger have to be somewhere in Paris at exactly the same time of day some time in 2014, if you do you win $1,000,000, if you don’t you die” (I like my hypotheticals extreme). So far well over 60% of people I’ve posed this question to have picked the same place, time and date. A lot more than that have picked at least 2 out of 3.
(and yes, there is usually some argument about how I enforce the “same time” rule, but I’m sure you get the gist)
John/22: The “death” thing makes it more likely I’ll pick a date near the end of the year. Is that what you intended?
In the movie “Contact”, the alien broadcast comes in on frequency “Hydrogen times pi”…. surely not a “natural” frequency, but one that is “naturally artificial” (‘Hydrogen’ here probably refers to a natural frequency related to a Hydrogen atom, namely 1420.40575177 MHz)
Some say, though, that ‘Hydrogen times 2\pi’ would be better. Even this assumes the aliens love geometry more than differential equations or number theory. Perhaps Hydrogen times e would be better, or Carbon times 1729? Or Silicon times (sqrt(5)+1)/2?
It may be hard to second-guess a completely alien intelligence. Could we, though, second-guess at least those intelligent life forms we have a decent chance of establishing communication with? What frequency should SETI really be paying attention to?
I was scrolling down to post something and noticed that Brian #6 beat me to it. So I guess we win, too.
Grabbing data from Google on the number of pages containing various numbers, it seems 1729 would only work for mathematicians, not the general public.
1725 108000000
1726 92000000
1727 92700000
1728 112000000
1729 91200000
1730 124000000
1731 93100000
1732 95400000
1733 95400000
*sigh*
John Faben 22. I like the Paris one. I have thought of my answer, but won’t post until a bit later to avoid contamninating the pool in case anyoine else wants to jump in.
Had to look up 137 – indeed it seems a good one for physicists. One could say that the number has a fine structure.
AIV #19. Given a range, the only logical numbers I can think of that would be apply to any range are the first and last numbers. For a range with an odd number of digits the middle one becomes another possibility.
@Phil: 1967
Ken B: We win!
@Phil: Actually, I’m a Montreal Canadiens fan myself. I grew up near Toronto, surrounded by you Mapel Leafs fans so I know the number …
Go Habs! (But Davie Keon rules.)
#28/29 is that when they were last any good?
@31: That was the last time the Montreal graciously allowed them to win the Stanley Cup, a final taste of victory before an eternity of failure and degredataion …
:) Sorry Phil, I said I was a Habs fan, and you what we’re like, especially when Toronto is mentioned!
#22 – the Paris one. I picked what I considerd the most iconic place – Eiffel Tower, most significant date for Paris – July 14th, and time was bit random – noon being the only one I could think of.
Not sure if 2014 is significant – couldn’t think of anything like London 2012 or Rio de Janeiro 2014. Another possibility would be Champs Elysees for the finish of the Tour de France.
#32 — hmm another thread devolving into jingoism…:)
Ken B: Thanks for 1967! I hadn’t realized you guys had been being gracious. I hope you similarly thank Kerry Fraser for 1993! And, of course, Dave Keon is one of the greatest ever from Quebec. Although, I do have a soft spot for Maurice Richard and that guy who hugged Paul Henderson. :)
Sorry, hijacking the thread. I’ll stop now. I bet that 90% of Leaf fans would choose “1967”, though.
For the Paris one, I’d consider choosing noon on Xmas day. That way, if the stranger chooses New Year’s Eve instead, we both get to live until December 25.
It would be 50/50 between that, and what Harold/33 said.
613
Joel: “613”
Is that the number of years to go before the Toronto Maple Leafs have a winning season?
“613” is the area code of the best place to watch the Leafs, because it peeves off the locals so much. Not something I’d choose as a Shelling Point, though.
I wonder if this game ties into “rational expectations” somehow? There seems something like a fixed point here in thoughts about he thinks I’ll think he thinks stuff. Like the one about the top of the Eiffel Tower at New Year’s. (Where you can toast another losing season for the Leafs.)
Stock Prices are schelling points, …. even for keynes, who says they are set like judges in a beauty contest.
If you are a San Francisco Giants fan you meet at the ballpark at 6:60pm (or as it is commonly referred: 7 pm) which is a perfect time to get into the ball game. The day would be Mays 6 (see what I did there?)
For musicians, in the range 1-100 our Schelling point might be 12 because 3^12 is fairly close to a power of 2. Thus, if you take the first 12 powers of 3 (0-11) and divide each term by 2 until it’s in the range 1-2 you get good ratios for a chromatic scale. This is why the western chromatic scale has 12 notes. For better ratios, multiply the first six terms by 2 and rearrange in ascending order, and then scale so that the first term equals 1. Then stick a 2 at the end. Like so:
1, 256/243, 9/8, 32/27, 81/64, 4/3, 729/512, 3/2, 128/81, 27/16, 16/9, 243/128, 2
The other most probable Schelling point is 7, the number of notes in most scales. Some say this has to do with how many notes the human brain retains in short-term memory.
6.02 E23
1776
911
Martin-2: Yes, I learned all this about chromatic scales fairly late in life. I should blog about it someday.
with all the econ and financial readers of this website I was surprised not to find:
What number between 1980 and 1990 would you pick?
what number between 1990 and 2000?
What date between 1980 and 1990?
what number between 2000 and 2010?
What number between 0 and 5? (including tenths, perhaps)
0 and 10?
0 and 3?
0 and 31?
Add your own, have fun.
I would have thought that mathematicians would go for “e” or “i” or pi.
I’d expect Americans to pick 1776 or 1789.
Aviators would pick the number associated with the aircraft they fly: from 172s up to 777s.
Tim
Steve, have you heard of an album called 12 Microtonal Etudes by Easley Blackwood? Each piece is in a different equal temperament from 13-24, and the jacket contains his notes on the strengths and weaknesses of each temperament. A good riddle for your readers might be what property 17 and 19 tone =temp have in common with 12 tone =temp that make them easy to use ;)
#48 Funny, I have just been looking at this stuff. I am not sure I have got it yet. After the octave, which are powers of 2, the next “whole number” ratios of frequency are fifths (3/2). We have 3 vibrations for 2 of the next note. These sound pleasant when sounded together. We can create a circle of fifths, where we move up by 3/2 each time. After 12 steps we are back to the first note (say C). This I think is what Martin-2 is saying. Without dividing by 2 we end up about 7 octaves higher, so we usually divide by powers of 2 to keep all the notes within the same octave range. When we arrange these in order, we get the familiar notes of the chromatic scale. Trouble is, when we get to the next octave, we are not quite at an octave of the original C because 3^12 is close, but not exactly the same as 2^19 – you end up with 2.027. Thus these very pleasing fifths do not quite fit into octaves. You have a choice, you can either keep to the exact ratio of 3/2, and then you have one interval that is discordant, or you adjust all the intervals to be the same. If you do the former, then some keys don’t work as you have a discordant interval, and playing together with instruments tuned to different keys is impossible. So we nearly always use equal temperament, where the fifths are not quite in the 3/2 ratio. This is why piano tuning is a skilled job.
So to the 17 and 19 question. With 12 note scale, A# and Bb are the same. With 19 notes most are different, except for two. Thus “normal” music maps onto the 19 note scale. I suspect it is something to do with priome numbers, and would be interested in an explanation.
I would love a blog about this music stuff (but will try to temper my enthusiasm while I wait)
For beginning mechanics, the Schelling point may be 710.
@ Tim F. #47
As it relates to knowing the years in which past events happened. The only question, I would expect the majority of Americans ( > 50%) to know is, “In what year did yesterday occur?”
#49 clearly, we should use a 53 note scale. Then, going through 84 fifths leads us to a note 31 octaves higher. I guess we wouldn’t call them ‘fifths’ any more.
#53 – I say, how did you manage to get that number for your comment? The 53 note scale is very very close to “pythagorean” tuning where the whole number ratios add up to octaves.http://en.wikipedia.org/wiki/53_equal_temperament
However, I think pianists would need either very thin fingers or very long arms!
Steve do we have to waterboard you for another post?! I’m going into withdrawal over here…
Bob Murphy: I promise to be very active next week!
A fine idea, Bob, but I’m just not sure that would be effective. Sure, we might get SOME post out of him. But he’d probably just spit out some random set theory theorem, fully knowing that it had long ago been undermined. Or he might quote p. 29 of The Big Questions, but leave out the fact that the quoted text reflected a misprint. And how could we verify the reliability of the circus-act videos he’d post?
So, without denigrating the many obvious advantages of your suggestion, I suspect it won’t prove practical in the long run.
@57: You make it sound more fun than Bob did though!