To read the comments on a post, or to add a comment, you can click on the title of that post.
Search:
-------------------------------------------------------------
Recent Posts
- Specialists
- Peter Calcagno!
- Trade War 2.0
- Price Theory and Applications — Errata
- Jamie Whyte!
- Ben Powell!
- Is This Too Cryptic?
- Harris’s 1922 Paths to Victory
- Degrees of Delusion
- Why We Need Price Theorists
- The Next Trump Cabinet
- The Next Democratic President
- Abortion and Public Policy
- The Tenth Time’s A Charm
- Specialized Markets
Archives
- March 2025
- February 2025
- October 2024
- August 2024
- July 2024
- June 2024
- May 2024
- April 2024
- March 2024
- February 2024
- December 2023
- November 2023
- September 2023
- August 2023
- June 2023
- April 2023
- March 2023
- December 2022
- November 2022
- September 2022
- July 2022
- June 2022
- May 2022
- April 2022
- January 2022
- October 2021
- August 2021
- July 2021
- June 2021
- May 2021
- April 2021
- March 2021
- January 2021
- December 2020
- November 2020
- October 2020
- September 2020
- August 2020
- July 2020
- June 2020
- May 2020
- April 2020
- March 2020
- February 2020
- January 2020
- December 2019
- October 2019
- August 2019
- June 2019
- May 2019
- April 2019
- March 2019
- January 2019
- December 2018
- November 2018
- October 2018
- September 2018
- August 2018
- July 2018
- April 2018
- March 2018
- February 2018
- January 2018
- December 2017
- November 2017
- October 2017
- September 2017
- June 2017
- May 2017
- March 2017
- February 2017
- January 2017
- November 2016
- October 2016
- September 2016
- August 2016
- July 2016
- June 2016
- May 2016
- April 2016
- March 2016
- February 2016
- January 2016
- December 2015
- November 2015
- October 2015
- September 2015
- August 2015
- July 2015
- June 2015
- May 2015
- March 2015
- January 2015
- December 2014
- November 2014
- October 2014
- September 2014
- July 2014
- June 2014
- May 2014
- April 2014
- March 2014
- February 2014
- January 2014
- December 2013
- November 2013
- October 2013
- September 2013
- August 2013
- July 2013
- June 2013
- May 2013
- April 2013
- March 2013
- February 2013
- January 2013
- December 2012
- November 2012
- October 2012
- September 2012
- August 2012
- July 2012
- June 2012
- May 2012
- April 2012
- March 2012
- February 2012
- January 2012
- December 2011
- November 2011
- October 2011
- September 2011
- August 2011
- July 2011
- June 2011
- May 2011
- April 2011
- March 2011
- February 2011
- January 2011
- December 2010
- November 2010
- October 2010
- September 2010
- August 2010
- July 2010
- June 2010
- May 2010
- April 2010
- March 2010
- February 2010
- January 2010
- December 2009
- November 2009
- October 2009
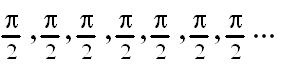



I’m no math expert, but I can tell this gonna be good.
*settles back with popcorn
I got a clue by googling “Sequence problems are pointless.”
That number is pretty close to 1/2. I’m betting it’s a instrument reading error since those are my favorite math problems.
Sir, you claim math created the universe. In light of integrals like these, I must ask you : who, then, created its sense of humor?
Damn! Missed it by THAT much…(holding two fingers close together)
Just to quibble, this is not an IQ test. ;-) In psych testing terms, an IQ test measures aptitude. This measures achievement (acquired knowledge). Or at the very least, this test requires a certain level of achievement in math before one can even begin to test the aptitude for problem solving.
@ Rowan:
I tend to agree with you.
The best definition of intelligence I have seen is: The ability to adapt.
If that is true, would not one faced with this problem do the research (adapt) in order to solve it?
Obviously, asking a 5th grader to solve it today would not be reasonable. Asking a 1st or 2nd year college student who wants a “complete/full/well-rounded” education, to solve it by year’s end, would be reasonable.
What say you?
The sequence approaches the motl ratio. Duh.
the answer you provide equates to pi/2 which is I guess the expected result. Why expressed in that particular way for the next in the sequence (rather than any other of the infinite ways it could be expressed) is well beyond me.
I’m just glad that it is pi/2 otherwise I will have gone crazy trying to work out why it’s a different value.
Shouldn’t we have discussed this on Pi Day?
Judging from the clues presented, I’d guess that an explanation is what comes next.
The solution provided is clearly satisfies Occam’s razor…. head meet wall
I would think that all else equal answering anything other than pi/2 should be considered an indication of lower intelligence than answering pi/2, the most parsimonious defensible answer lacking other information (note, ‘this is a calculus problem’ does not add information).
There may be a social-intelligence dimension here (i.e. if you need to impress someone such as a potential employer, or blog readers, you intuit that pi/2 is a ‘bad’ answer), but I don’t think that figures into IQ?
no. the answer is 34.
starting at about 2:20 minutes.
The thing that comes next is a comma. Period.
@martin: Good video – but you really should supply the right polynomial for 34 to fit with THIS sequence.
The eighth item in the sequence is “ice cream” and I didn’t need any calculus. I just wrote out the obvious rule for the nth item a(n):
a(n)= (“ice cream”-π/2)*(n-1)*(n-2)*(n-3)*(n-4)*(n-5)*(n-6)*(n-7)/(n-1)! + π/2
and after seven half-π’s, I ended up with ice cream.
Well, I guess I’m smart enough to guess that what’s next is NOT that symbol over 2. Do I win?
Because 935615849440640907310521750000/467807924713440738696537864469=2
@marek: Yes, that number is the motl limit of a sequence of 2s.
What’s a motl limit?
@ Boria:
Even if you have a test to see how well one can adapt it still is about adapting over the short time period of the test.
Possibly acquired knowledge is a measure of adaptation over several years.
So either way it still tests a very specific intelligence.
The answer is not equal to pi/2, even though it rounds it to that on the calculator when you divide, but when you subtract twice the numerator from the denominator you get a big number, so it’s more like 0.49999999999999*pi.
@ Marek: 467807924713440738696537864469 * 2 ends with an 8
@martin, @jens:
The unique 7th degree polynomial which evaluates to π/2 at x = {0, 1, 2, 3, 4, 5, 6} but is N at x = 7 is:
-π(x-1)(x-2)(x-3)(x-4)(x-5)(x-6)(x-7)/10080
+π(x-0)(x-2)(x-3)(x-4)(x-5)(x-6)(x-7)/1440
-π(x-0)(x-1)(x-3)(x-4)(x-5)(x-6)(x-7)/480
+π(x-0)(x-1)(x-2)(x-4)(x-5)(x-6)(x-7)/288
-π(x-0)(x-1)(x-2)(x-3)(x-5)(x-6)(x-7)/288
+π(x-0)(x-1)(x-2)(x-3)(x-4)(x-6)(x-7)/480
-π(x-0)(x-1)(x-2)(x-3)(x-4)(x-5)(x-7)/1440
+N(x-0)(x-1)(x-2)(x-3)(x-4)(x-5)(x-6)/5040
You can use this technique to justify any number as being the next number in the sequence. The video argues that 2*n is a simpler explanation to the sequence {2, 4, 6, 8} since it has a lower degree than the other polynomial he uses, but I disagree. I think my answer is simpler since it can find a polynomial for any sequence, not just the one special sequence {2, 4, 6, 8}.
This technique is called the Lagrange interpolation formula. It was used by Karl E. Linderholm to trivialize all questions of the form “What is the next number in this sequence?” in his great classic, Mathematics Made Difficult.
Since you posed the question as an IQ test my guess at what comes next is a comma. Pretty sure that is correct.
Tim
@marek: The Motl Lemma was discussed a great deal on this blog about a year ago. Googling will turn up reams of stuff. The short answer is this: the Motl Lemma states that in any sequence which converges to 1/2 all terms are equal to 1/2. Hence that term is also equal to 1/2 and fits the pattern very well. Steve has a summary here http://www.thebigquestions.com/2011/12/30/turning-the-crank-the-year-in-review/
The original problem asked, “What comes next in the following series?”
As I understand English, “what” does not mean “what number” and, as I understand math, the “series” is more properly called a sequence.
Clearly my first answer: “comma” is most appropriate.
The comment regarding calculus is ambiguous, as calculus has several different meanings, and instead of “ice cream,” I could have submitted “tartar.”
It seems y’all are answering a question not posed, namely:
“Assuming the missing comma to be implicit, what number most nicely follows in the given mathematical sequence.”
@Jon Shea
re: “I think my answer is simpler since it can find a polynomial for any sequence, not just the one special sequence {2, 4, 6, 8}.”
if the problem is to solve many (say 1000) such problems, then yes, the general algorithm/solution that solves all problems will be simpler than the sum of all the individual algorithms/solutions.
But, for the simple case 2,4,6,8 , 2 * n is simpler than any gereralized solution.
if by simple we mean one of the precise definitions given in the video …
Whoever made the answer key is terrible at simplifying fractions. Were I a math teacher I would only give half credit.
I’m not looking to sec controversy or go off on a tangent but not asking a trigonometry question so close to pi day seems like a sin.
I followed Roger Schalafly. This is a a very Interesting example. Landsburg’s hint is kinda pointless. It is impossible for anyone to figure this out unless you noticed the sequence in your work or read about it somewhere.
Just like the meaning of life the answer is 42. This is the sequence of solutions of:
(x – 42)*(x – pi/2)^7
Surely this is obvious?
And you can drop the three dots Landsberg. That gives people the false impression this sequence is infinite. And you need to learn the difference between a sequence and a series.
Tsk…
Clearly, the answer given is not exactly pi/2. A simple two-line
REXX program[1] gives the answer to the division as
0.4999999999926468593249718283124405644109 (…)
The official “answer” to the next-in-series is supplied as a
graphic, seemingly to make it more difficult to automate the result.
As to why this inaccurate approximation of 1/2 would be more correct
than 1/2, I have no idea.
[1] the program is:
numeric digits 40
say 467807924713440738696537864469/935615849440640907310521750000
@Will A: “I’m not looking to sec controversy ”
I see no sin of it. Otherwise I’d cosh you on the head. Assuming I cot you.
As was said, this is not an IQ test. There have been no studies showing how scores on this test correlate with those on other IQ tests or covary with general achievement or other measures of intelligence (i.e., it has not been validated). Also, the limited number of items on the test greatly reduces its utility in differentiating between various IQ levels, which is one of the primary reason for measuring intelligence. Additionally, an IQ is derived from scores on several different subtests which measure various domains (e.g., verbal skills, abstract reasoning, working memory). At best, this test would provide information relevant to one specific domain. Even then, however, a person might get this question wrong, but still score high on the construct measured by this question were other questions available to measure this construct.
Anyone who thought this was an IQ test failed the IQ test.
This was an amusing teaser for a result to be named latter. Steve saw a result proing that a series of caluculus questions has a simple answer, 1/2, until a certain point and then this sudden shift to an answer amazingly close to 1/2. Steve’s post is a jesting way to highlight this remarkable result(which we do not know yet).
Sorry to spoil the joke Steve but after my boner and neutrinos jokes I feel entitled :)
A comma, surely.
Then the \ldots.
(sorry)
I plugged the numbers into excel and got pi/2, but it probably rounded off somewhere.
It turns out that when I have Google in front of me, I have a high IQ.