 Bertrand Russell, that most rational of men, was nonetheless plagued by intermittent depression and the occasional nightmare. Including this one, as reported by Russell’s confidante, the mathematician G.H. Hardy:
Bertrand Russell, that most rational of men, was nonetheless plagued by intermittent depression and the occasional nightmare. Including this one, as reported by Russell’s confidante, the mathematician G.H. Hardy:
[Russell] was in the top floor of the University Library, about A.D. 2100. A library assistant was going round the shelves carrying an enormous bucket, taking down books, glancing at them, restoring them to the shelves or dumping them into the bucket. At last he came to three large volumes which Russell could recognize as the last surviving copy of Principia Mathematica. He took down one of the volumes, turned over a few pages, seemed puzzled for a moment by the curious symbolism, closed the volume, balanced it in his hand and hesitated….
Principia Mathematica, to which Russell had devoted ten years of his life, was his (and co-author Alfred North Whitehead‘s) audacious and ultimately futile attempt to reduce all of mathematics to pure logic. It is a failure that enabled some of the great successes of 20th century mathematics. And — the first volume having been published in December, 1910 — this is its 100th birthday.
Having determined to write the Principia ten years earlier in 1900, Russell was at first stymied by his discovery of the famous paradox that now bears his name: Consider the set of all those sets that don’t contain themselves. Call this set R. Does R contain itself? If so, it belongs to the set of all sets that don’t contain themselves, and therefore does not contain itself. Does it fail to contain itself? If so, it fails to belong to the set of all sets that don’t contain themselves, and therefore contains itself. Either way, something’s screwy.
The Russell Paradox suggested that set theory was too shaky a foundation on which to build the edifice of mathematics, and Russell realized that the first thing he needed to do was shore up that foundation — a task that led to months, and then years, of staring at blank pages with no idea of how to proceed:
At first I supposed that I should be able to overcome the contradiction quite easily, and that probably there was some trivial error in the reasoning. Gradually, however, it became clear that this was not the case…It seemed unworthy of a grown man to spend his time on such trivialities, but what was I to do?
Russell’s ultimate solution was his Theory of Types, which (some would say artificially) limits the ways in which you’re allowed to define a set — and in particular prohibits you from defining the set R in the first place. It was clunky and ad hoc and many found it profoundly unsatisfactory, but it worked.
There were other problems, too. Russell wanted to derive all of mathematics from pure logic, but there was one mathematical fact that defied his every effort — namely the fact that there are infinitely many natural numbers. Surely this is a mathematical fact, but does it follow from purely logical considerations — or is it just something that happens to be true without being logically necessary? In the end, Russell punted, taking the infinitude of the natural numbers as an (essentially non-logical) axiom. Here was the first hint that maybe mathematics is more than just logic after all.
Once he had solved these problems — or, on a less charitable interpretation, decided how he was going to weasel his way around them — Russell spent the years from 1907 through 1910 working on the manuscript, ten to twelve hours a day, eight months a year. He worked, of course, with the technology of the time, with all of its attendant dangers and inconveniences:
The manuscript became more and more vast, and every time that I went out for a walk I used to be afraid that the house would catch fire and the manuscript get burnt up. It was not, of course, the sort of manuscript that could be typed, or even copied.
When we finally took it to the University Press, it was so large that we had to hire an old four-wheeler for the purpose. Even then our difficulties were not at an end.
The University Press estimated that there would be a loss of £600 on the book, and while the syndics were willing to bear a loss of £300, they did not feel that they could go above this figure. The Royal Society very generously contributed £200, and the remaining £100 we had to find ourselves. We thus earned minus £50 each for ten years’ work. This beats the record of Paradise Lost.
The first edition of Volume I ran to 750 copies. Volumes II and III, published in 1912 and 1913, ran to 500 copies each.
Aside from dissatisfaction with the Theory of Types and the Axiom of Infinity, there were a couple of other nagging questions left unsettled, though. First: Could all of mathematics be derived from Russell and Whitehead’s logical system? Surely some of it could (though not always easily — R and W notoriously required hundreds of pages to reach the conclusion that 1+1=2) — but could all? And second: Could the Russell/Whitehead system be proven to be free of logical contradictions? The Russell Paradox had been excised by the Theory of Types, but could one exclude the possibility of other paradoxes lurking in the background?
Russell was surely hopeful on both counts. Kurt Godel, the logician of the millennium and the man who would dash those hopes, was four years old in 1910.
I venture to guess that nobody has ever read the three large volumes of Principia Mathematica, a typical page of which looks about like this:
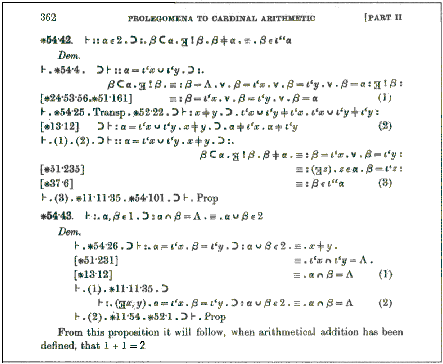
But, for having set the agenda for (at least) a century of research into the foundations of mathematics, I am sure it will still be celebrated in the A.D. 2100 of Lord Russell’s nightmare.
[RELATED POST: Principia Mathematica: The Comic Book”]



can you give an example of a set that does contain itself?
Dave: Naively, “the set of all sets” (if there were such a thing) would contain itself, since it is a set, and therefore belongs to the set of all sets.
Nowadays, we don’t allow ourselves to talk about things as big as “the set of all sets”, but in the early 20th century, people formed sets with abandon and examples like this would have been common.
Can you give some idea of what they were doing in the hundreds of pages to show that 1+1=2?
I look at that page and the first thing I think is: Damn, copy-editing that must have been a bitch. I also wonder how many pages the errata ran to.
An excellent summary Steve, but non-math types might not be aware just how serious a problem this is. In formal abstract mathematics is common to (try to) define the natural numbers in terms of sets. ) is the empty set (call it @), 1 is the set containing the empty set, {@} — which is not an empty set, etc. With the usual ZF axioms of set theory you can prove this structure satisfies the Peano Axioms for natural numbers. Pretty much everything in math is built from sets. So the inadequacy of set theory as a foundation — which most mathematicians blithely and happliy ignore — is a very serious problem. As Steve has shown in other posts though this strongly affects what the real numbers are actually like. Like the theorem from last week that you can predict the future with essentially perfect accuracy, which follows from one baseless assumption (the name for those is axiom) which we is generally made not specifically about real numbers but about how to plug Russell’s hole.
Ken B: Thanks for adding this.
“Consider the set of all those sets that don’t contain themselves. Call this set R. Does R contain itself? If so, it belongs to the set of all sets that don’t contain themselves, and therefore does not contain itself. Does it fail to contain itself? If so, it fails to belong to the set of all sets that don’t contain themselves, and therefore contains itself. Either way, something’s screwy.”
Maybe I am just confusing myself here, but shouldn´t it be the other way around?
If R contains itself, then it fails to belong to the set of all sets that don´t contain themselves and cannot contain itself. On the other hand if R does not contain itself, then it does belong to the set of all sets that don´t contain themselves, and therefore contains itself.
Or did I misunderstand something?
“Consider the set of all those sets that don’t contain themselves. Call this set R. Does R contain itself? If so, it belongs to the set of all sets that don’t contain themselves, and therefore does not contain itself. Does it fail to contain itself? If so, it fails to belong to the set of all sets that don’t contain themselves, and therefore contains itself. Either way, something’s screwy.”
Maybe I am just confusing myself here, but shouldn´t it be the other way around?
If R contains itself, then it fails to belong to the set of all sets that don´t contain themselves and cannot contain itself. On the other hand if R does not contain itself, then it does belong to the set of all sets that don´t contain themselves, and therefore contains itself.
Or did I misunderstand something?
@ obi
What you have is a contradiction in either case (R is both contained and not contained in itself) so you can really go about it in either way.
In Steven’s explanation, we have that R is in R, the set of all sets that don’t contain themselves, so R cannot contain itself and R cannot be in R.
Your logic pushes the contradiction in the other direction: R is in R, so R contains itself, and therefore cannot be in the set of all sets that fail to contain themselves, R.
“balanced it in his hand and hesitated…” Is that when he woke up?
Obi:
You wrote:
“If R contains itself, then it fails to belong to the set of all sets that don´t contain themselves ”
I am not sure where you’re getting this. R is the set of all sets that DON’T contain themselves. If R contains itself, then R belongs to R, which means that R DOES belong to the set of all sets that don’t contain themselves.
You say that Russell’s work was a failure, but your only justification is that it did not do what others naively thought that it might do. W & R did succeed in giving an axiomatic foundation for set theory and mathematics. The books are not read today because they have been superseded by improved versions, but they were still successes.
Roger Schlafly: But W&R’s stated goal was not just to provide an axiomatic foundation for set theory and mathematics — it was to provide a foundation in which all of the axioms were logically necessary. The axiom of infinity arguably fails to meet that criterion.
In addition, of course, W&R wanted a system from which it was possible to derive all of the truths of arithmetic, not just a large number of them. We know now that this goal was doomed to failure from the start.
I agree with n+1, Obi is just showing the contradiction in the other direction. When Obi analyzes the statement “R contains itself” he explains the consequences for the “R” part of the statement. When Steve analyzes that statement, he explains the consequences for the “itself” part of the statement.
Obi’s statement, “If R contains itself, then it fails to belong to the set of all sets that don´t contain themselves ” is tautological: It is like saying “If X does Y, then it fails to belong to the set of things that do not do Y.” Obi moves from this fact to the contradiction.
The axiom of infinity is logically necessary. If W&R said that, then they were correct.
Was Alexander Grothendieck a failure because he had a program to prove all the theorems of algebraic geometry, but he did not prove all those theorems?
@Roger:
Steve did not say Russell failed full stop, or that his work in toto was a failure. He said that Russell failed in reducing math to pure logic, and that he did. Because it cannot be done.
@ Prof. Landsburg,
I think I got a little confused with the fact that in your example saying “R” and saying “the set of all sets that don´t contain themselves” is of course equivalent.
@ n+1,
big thanks for your explanation. It really helped me to see what was missing in my reasoning.
Maybe this is a good example why a typical page in the Principia Mathematica looks the way it does, because the symbolical representation of the paradox on it´s wikipedia page is in my opinion a lot less confusing than a verbal explanation.
Roger Schlafly: Grothendieck certainly failed to realize his vision of a fully motivic basis for algebraic geometry. This, of course, doesn’t contradict the fact that he was the greatest mathematician of his century, and quite possibly of all time.
@Steve:
I certainly make enough typos and spelling mistakes, so maybe I shouldn’t point this out but I do think you misspelt G-A-U-S-S in you latest comment. :>
So Russell was a failure in the same sense that the greatest mathematician of the century was a failure. I wish that I could also be such a failure!
Roger:
I wish that I could also be such a failure!
Me too!
***** Probably a stupid question warning *****
In regards to the statement:
The Russell Paradox suggested that set theory was too shaky a foundation on which to build the edifice of mathematics.
Does anyone know if work has been done to show whether or not any problem in Logic Theory can be reduced to any problem in Set Theory?
De Morgan’s Laws would be an example. What about paradoxes?
Using a probably very bad metaphor from the concept of NP Completeness. Does the paradox “This sentence is not provable” reduce to “Consider the set of all those sets that don’t contain themselves. Call this set R. Does R contain itself?” or some other paradox from set theory?
If every problem can be reduced, then we could finally get logic out of the hands of philosophers and into the hands of Mathematicians and Computer Scientists where it belongs.
We could probably do this in the similar way that Russell was trying to get mathematics out of the hands of Mathematicians and into the hands of philosophers where he thought it belonged.
@Ken B: Your point is that Russell failed to do something that cannot be done. The same could be said about all people and all work. It all fails to do the impossible.
Roger: But it cannot be said of all people and all work that their *goals* are impossible.
i thought about this a bit last night and perhaps managed to answer my own question and create some new ones in the process.
{the set of all sentence fragments that start with the letter ‘t’}
which led me to believe that there is some circular reasoning going on here.
what really threw me for a loop was when i realized that this set was clearly finite.
then i fell asleep. =] happy holidays folks!
Steve: Russell is just as famous for having a goal of world peace. Now that is a goal that is impossible! But where does PM state an impossible goal? I am looking at the introduction to it, and W&R state 3 goals in the first paragraph: (1) to effect the greatest analysis with the fewest axioms, (2) to use precise and convenient notation, and (3) to solve the paradoxes of set theory. It seems to me that they achieved these goals admirably.
They say a couple of pages later that an object of the work is “the complete enumeration of all the ideas and steps in reasoning employed in mathematics”. Do you have some complaint about this? They do not describe all of mathematics, but they do a good job of capturing the reasoning in the foundations of math.
@ Dave:
I’m trying understand how this is a finite set. The following sentence fragment seem to be in the set also:
“The even number 2”
“The even number 4”
“The even number 6”
. . .
This seems like an infinite set to me.
@ Roger:
I haven’t read the PM, so I don’t know if it effected the greatest analysis with the fewest axioms.
Did Russell prove that it was impossible for anyone to do greater analysis with the same number or fewer number of axioms? Or did he leave it to the reader to prove on his own?
Will A: W&R did not claim perfection. They left it to others to improve their work, and others did improve it.
i guess i didnt think hard enough.
i was under the mistaken impression that since there seems to be a finite number of words that there would be a finite number of combinations of those words. maybe if we only had one word for numbers greater than four, we would be happier.
@Roger:
I just think you’re committing a logical fallacy here. Steve said Russell failed to achieve goal X. You infer from that that Steve called Russell a failure. From “Russell failed to achieve X” you infer “Russel failed to achieve” That’s just wrong.
Steve did not diss Russell, he noted his stated goal was later proved impossible.
Ken B: No, Steve did not do that. He misstated Russell’s goals and what Russell accomplished. W&R succeeded in their goals.